A comparative study of ptdf based - Methods to determine transmission usage allocation for bilateral transactions in power markets

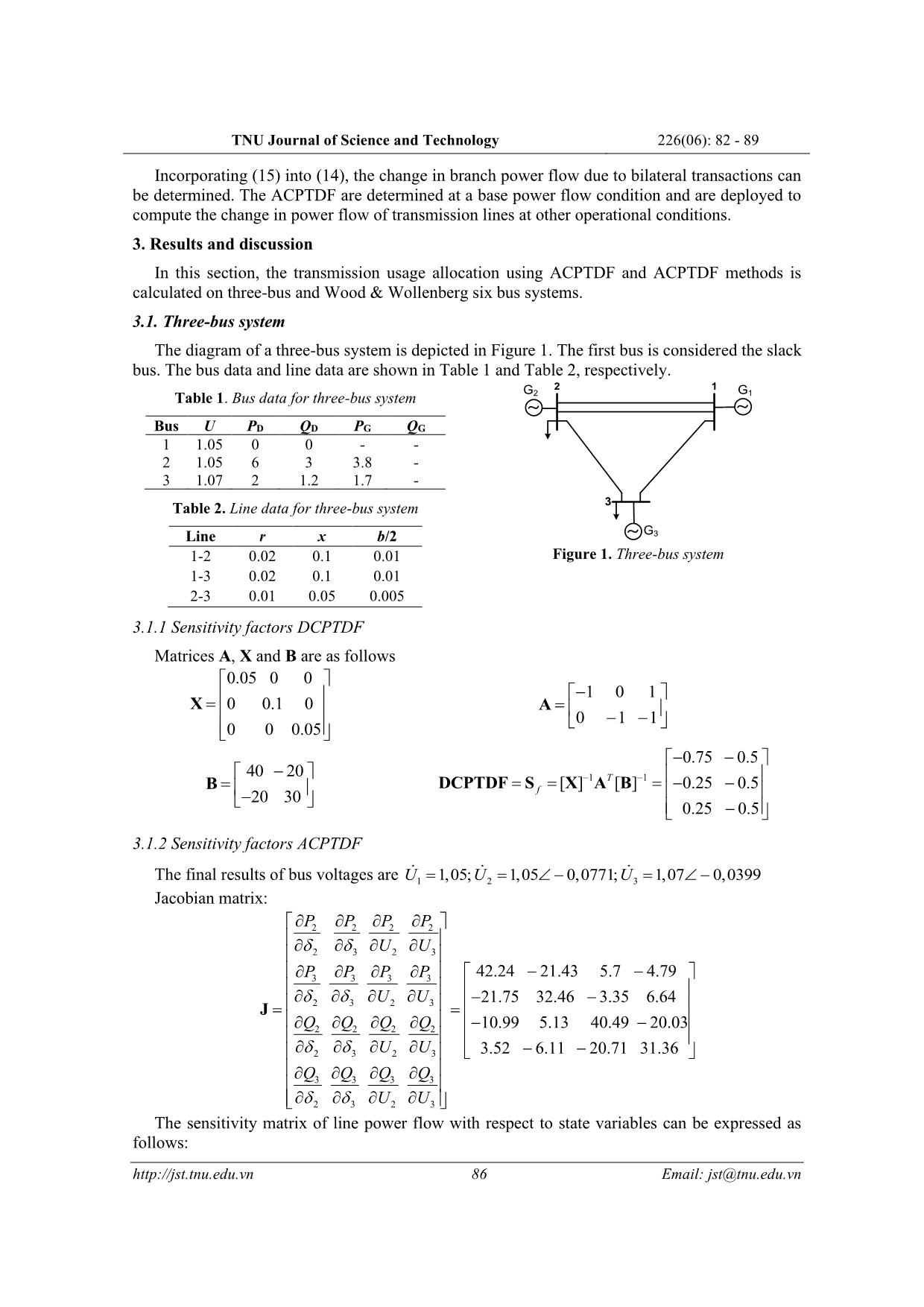
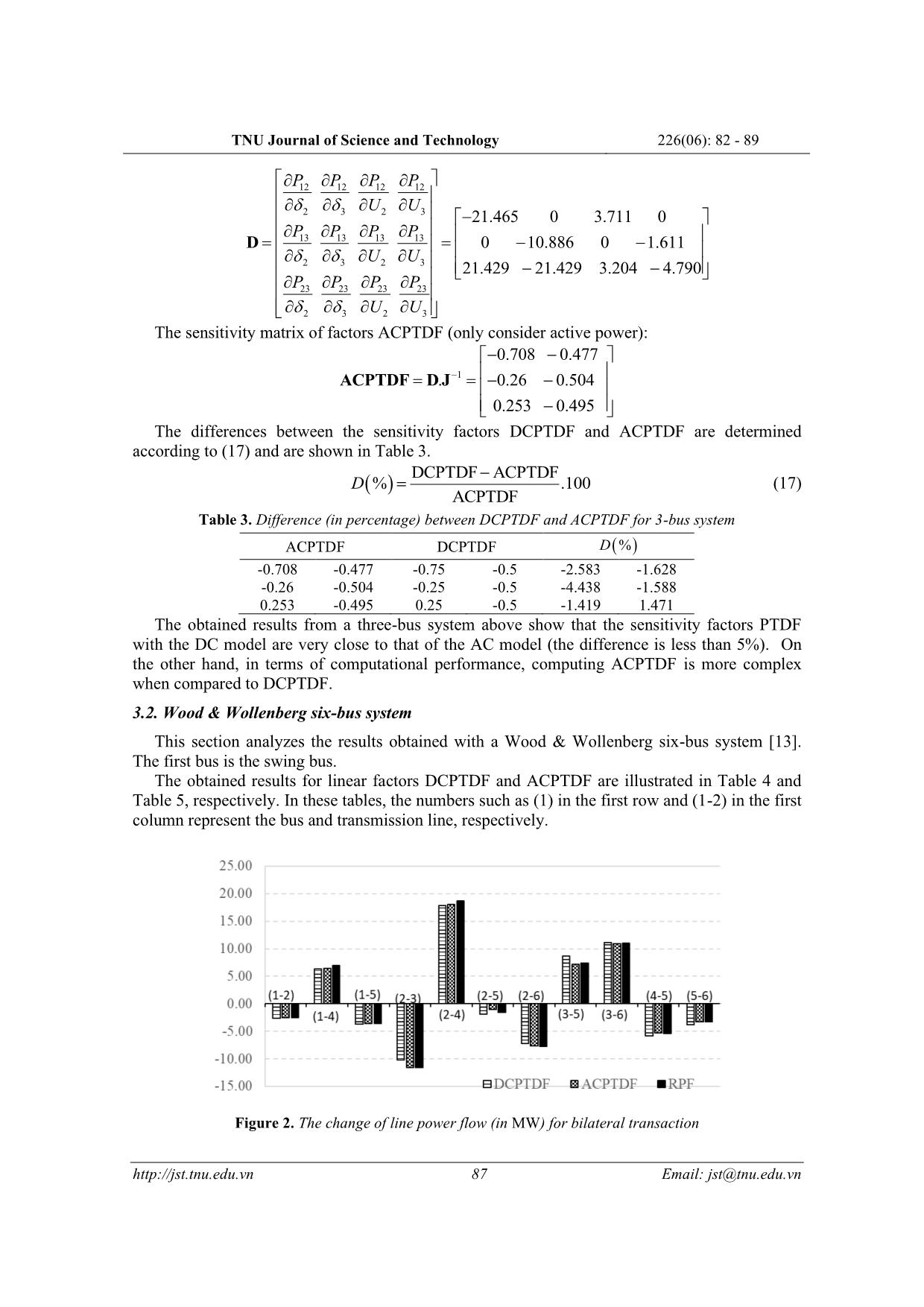
TNU Journal of Science and Technology 226(06): 82 - 89 
 82 Email: jst@tnu.edu.vn 
A COMPARATIVE STUDY OF PTDF BASED-METHODS 
TO DETERMINE TRANSMISSION USAGE ALLOCATION 
FOR BILATERAL TRANSACTIONS IN POWER MARKETS 
Pham Nang Van* 
School of Electrical Engineering - Hanoi University of Science and Technology 
ARTICLE INFO ABSTRACT 
Received: 22/02/2021 The electricity industry has been deregulated with the aim of 
producing competition among power market participants, which 
would result in considerable difficulties for power system operators. 
One of these challenges is that power system operators need to give 
participants nondiscriminatory access to transmission usage. In this 
study, the transacted power flow in transmission networks for bilateral 
transactions deploying methods based Power Transfer Distribution 
Factor (PTDF) was analyzed and compared. The Power Transfer 
Distribution Factor can be classified as DCPTDF and ACPTDF. The 
results from these approaches using a three bus and Wood & 
Wollenberg six bus systems were elaborately computed and 
compared. The comparison shows that leveraging factors ACPTDF to 
allocate transmission usage is more exact but complicated than the 
techniques based factors DCPTDF. The comparative study in this 
paper provides a comprehensive evaluation that can support power 
system operators to achieve nondiscrimination in power trade. 
Revised: 28/05/2021 
Published: 31/5/2021 
KEYWORDS 
Power markets 
Power transfer distribution factor 
(PTDF) 
ACPTDF 
Transmission usage allocation 
Bilateral transactions 
SO SÁNH CÁC PHƯƠNG PHÁP SỬ DỤNG HỆ SỐ PTDF 
ĐỂ PHÂN BỔ CÔNG SUẤT TRÊN LƯỚI TRUYỀN TẢI 
CỦA CÁC GIAO DỊCH SONG PHƯƠNG TRONG THỊ TRƯỜNG ĐIỆN 
Phạm Năng Văn 
Viện Điện - Trường Đại học Bách khoa Hà Nội 
THÔNG TIN BÀI BÁO TÓM TẮT 
Ngày nhận bài: 22/02/2021 Ngành điện đã và đang tái cấu trúc với mục đích tạo ra sự cạnh tranh 
giữa những người tham gia thị trường điện. Sự tái cấu trúc này dẫn 
đến những khó khăn đáng kể cho các đơn vị vận hành hệ thống điện. 
Một trong những khó khăn này là các đơn vị vận hành hệ thống điện 
cần cung cấp cho đơn vị tham gia thị trường quyền sử dụng lưới điện 
truyền tải một cách công bằng. Trong nghiên cứu này, phân bố công 
suất trên lưới điện truyền tải của các giao dịch song phương được 
phân tích và so sánh sử dụng hệ số phân bố truyền tải công suất 
(PTDF). Hệ số phân bố truyền tải công suất được phân loại thành 
DCPTDF và ACPTDF. Kết quả tính toán từ cả hai tiếp cận này sử 
dụng các hệ thống điện 3 nút và 6 nút Wood & Wollenberg được 
phân tích và so sánh. Sự so sánh cho thấy phân bổ công suất trên lưới 
truyền tải của các giao dịch song phương khi áp dụng hệ số ACPTDF 
chính xác hơn nhưng phức tạp hơn so với sử dụng hệ số DCPTDF. 
Kết quả nghiên cứu trong bài báo này cung cấp sự đánh giá chi tiết để 
hỗ trợ các đơn vị vận hành hệ thống điện đạt được sự công bằng 
trong hoạt động thị trường điện. 
Ngày hoàn thiện: 28/05/2021 
Ngày đăng: 31/5/2021 
TỪ KHÓA 
Thị trường điện 
Hệ số phân bố truyền tải công suất 
(PTDF) 
ACPTDF 
Phân bổ sử dụng truyền tải 
Giao dịch song phương 
DOI: https://doi.org/10.34238/tnu-jst.4019 
Email: van.phamnang@hust.edu.vn 
TNU Journal of Science and Technology 226(06): 82 - 89 
 83 Email: jst@tnu.edu.vn 
1. Introduction 
Power deregulation aims at creating competitive electricity markets. In these markets, 
consumers have options to purchase electricity from various producers. Therefore, efficient 
transmission usage allocation needs to be studied to implement power transfer between diverse 
consumers and producers. Determining the extent of transmission service use aims to (1) 
equitably allocate transmission costs to those participating in the power grids and (2) calculate 
loss indicators to operate transmission grids more reliably and efficiently. Furthermore, fair 
transmission service is also essential in giving signals to the marketplace for long-term 
investment. Applying power flow methods such as Newton-Raphson, Fast Decoupled Power 
Flow (FDPF) to accurately compute the transmission usage level of market participants is 
complicated and time-consuming [1]. 
There are some effective approaches developed to deal with the problem of transmission 
usage allocation. In [2], the author proposed a topology-based approach to determine the share of 
a particular generator or demand in the power flow of each line branch. The paper [3] developed 
another technique based on concepts such as the domain of a generator, commons, links and state 
graph. The procedure using graph theory was put forward in [4] to determine injection factors of 
individual generators to line branch flows and withdrawal factors of individual demands from 
line flows. Authors in [5] introduced an AC power transfer distribution factor (PTDF)-based 
method to allocate the real power flow on transmission lines. 
PTDFs are coefficients of the linea ... rch has made major contributions as follow: 
• Rigorously present a step-by-step procedure to calculate ACPTDF using a three-bus system; 
• Compare the findings of transmission usage allocation from both kinds of sensitivity factors 
PTDF. 
The paper is structured into four sections. Section 2 presents the Newton-Raphson method, 
formulations of DCPTDF and ACPTDF. Numerical results and discussions using three-bus and 
Wood & Wollenberg six-bus systems are given in Section 3, and the conclusions are inferred in 
Section 4. 
2. Methodology 
2.1. Newton-Raphson method 
This section addresses the formulation and Newton-Raphson solution to the power flow 
problem. The complex current injected to the bus i is expressed as follow: 
1
1,2,...,
n
i ik k
k
I Y U i N
=
= = (1) 
TNU Journal of Science and Technology 226(06): 82 - 89 
 84 Email: jst@tnu.edu.vn 
where kU is the complex voltage at node k; ik ik ikY G jB= + is the ikth element of the 
admittance matrix, and N is the total nodes of the power system with 
GN generator buses and 
DN load nodes. Bus 1 is chosen as the voltage reference bus. 
The complex power at ith bus: 
*
* *
1 1
n n
i i ik k i ik k
k k
S U Y U U Y U i
= =
= =  
  (2) 
Using rectangular coordinates for elements of the admittance matrix and polar form for 
voltages leads to: 
 ( )ik
1
( ) cos sin
n
i i k ik ik ik
k
S U U G jB j i 
=
= − +  (3) 
where 
ik is the difference between the phase angles of nodes i and k; ,i kU U is the voltage 
magnitude at bus i and k, respectively. 
Split (3) into 2n real equations: 
1
1
( cos sin )
( sin cos )
n
i i k ik ik ik ik
k
n
i i k ik ik ik ik
k
P U U G B
Q U U G B
 
 
=
=
= + 
 = −


 (4) 
The Newton-Raphson (NR) successively improves unknown values through the first-order 
approximation of the involved non-linear equations. According to NR method, with initial values, 
corrections are obtained by solving the linear equation system: 
( )
( )
( )r r
r 
= 
P δ
J
Q U
 (5) 
where J is the Jacobian matrix; ΔP and ΔQ are the difference between the specified power 
and the power computed with the most recent values. 
The iteration continues until all the mismatch vector of power in absolute value lower than the 
pre-specified tolerance. 
2.2. DC Power Flow 
This DC power flow (DCPF) method is developed with assumptions as follows: 
• the voltage magnitude at all buses is equal to 1; 
• the phase angle difference corresponding to adjacent buses are small; 
• series resistance is ignored. 
With these hypotheses, the real power flow is simplified to 
 ( )
1
ik i k
ik
P
x
 = − (6) 
where
ikx is the reactance of ikth branch. 
The linear relationship between nodal power injection and voltage angle, according to the 
DCPF method, is written as follows: 
 ( )
1
i ik i k
k k ik
P P
x
 = = −  (7) 
2.3. DCPTDF formulation 
Equation (7) can be rewritten in matrix form as P = Bδ . The relationship between branch 
power flow fP and nodal injected power P can be obtained by eliminating phase angles: 
TNU Journal of Science and Technology 226(06): 82 - 89 
 85 Email: jst@tnu.edu.vn 
1
1 1
T T
f f
T
f f f
−
− −
 = = 
 = = = 
A δ XP P X A δ
P AP P X A B P S P
 (8) 
where A is the reduced branch-to-node incidence matrix omitting the swing node, X is a 
diagonal matrix with elements of branch reactances, Sf is the matrix of sensitivities between 
branch power flows and nodal powers. 
Then, the power flow on the branches after a change in nodal power can be computed as 
follows: 
 ( )0 0f f f f f= + − = P P S P P P S P (9) 
Power transfer distribution factor (PTDF) is defined as a power flow increase on the mn line 
when the power injected in ith node increases by 1 MW 
i
mn m n
mn i mn i
i mn
P
PTDF S
P x
 
− −
 − 
= = =
 (10) 
The PTDF above depends solely on the structure of electrical networks; therefore, these 
factors can be calculated off-line using sparse matrix techniques. However, the PTDF depends on 
the location of the voltage reference node. 
2.4. ACPTDF formulation 
The sensitivity factors ACPTDF are applied to determine a change in the power flow of 
branches after changing in power transactions at different operating states from the sensitivity 
values of the Jacobian matrix in the Newton-Raphson algorithm. 
Consider a bilateral transaction Pt between the seller at bus i and the buyer at bus j. 
Furthermore, consider a line mn which is connected between nodes m and n and transfers the part 
of the transacted power. When the active power contract ( ,t i jP− ) between the above market 
participants is changed, the variation in the power amount of transmission line mn (
mnP ) is 
calculated as 
 , ,ACPTDFmn mn i j t i jP P− − = (11) 
Equation (5) can be expanded as (12). The change in power flow of line mn can be determined 
using sensitivity analysis as (13). 
1
2 1 2 1( )G G D G G D
T T
N N N N N N N NU U P P Q Q 
−
+ + + +
 = J (12) 
2 1
2 1
... ...
G G D
G G D
T
mn mn mn mn
mn N N N N
n N N N
P P P P
P U U
U U
 
 
+ +
+ +
    
 =     
 (13) 
Substituting equation (12) in equation (13), the change in power flow of line mn can be 
calculated as equation (14). 
1
2 1
2 1
... ... ( )
G G D
G G D
T
mn mn mn mn
mn N N N N
N N N N
P P P P
P P P Q Q
U U 
−
+ +
+ +
    
 =     
J (14) 
A bilateral contract is defined by a tuple (t, i, j, Pt), in which t is the contract number, i and j 
are the seller and buyer buses, respectively, and Pt is the transacted power. For a bilateral 
transaction t: 
 ; ; 0; 0 2,..., ; ,i t j t k kP P P P P Q k N k i j = + = − = = = (15) 
The linear factors ACPTDF can be obtained from the following equation: 
1
,
2 1
ACPTDF ... ... ( )
G G D
mn mn mn mn
mn i j
N N N N
P P P P
U U 
−
−
+ +
    
= 
     
J (16) 
TNU Journal of Science and Technology 226(06): 82 - 89 
 86 Email: jst@tnu.edu.vn 
Incorporating (15) into (14), the change in branch power flow due to bilateral transactions can 
be determined. The ACPTDF are determined at a base power flow condition and are deployed to 
compute the change in power flow of transmission lines at other operational conditions. 
3. Results and discussion 
In this section, the transmission usage allocation using ACPTDF and ACPTDF methods is 
calculated on three-bus and Wood & Wollenberg six bus systems. 
3.1. Three-bus system 
The diagram of a three-bus system is depicted in Figure 1. The first bus is considered the slack 
bus. The bus data and line data are shown in Table 1 and Table 2, respectively. 
Table 1. Bus data for three-bus system 
Bus U PD QD PG QG 
1 1.05 0 0 - - 
2 1.05 6 3 3.8 - 
3 1.07 2 1.2 1.7 - 
Table 2. Line data for three-bus system 
Line r x b/2 
1-2 0.02 0.1 0.01 
1-3 0.02 0.1 0.01 
2-3 0.01 0.05 0.005 
2 1
3
G1G2
G3 
Figure 1. Three-bus system 
3.1.1 Sensitivity factors DCPTDF 
Matrices A, X and B are as follows 
0.05 0 0
0 0.1 0
0 0 0.05
 =
X 
1 0 1
0 1 1
− 
= 
− − 
A 
40 20
20 30
− 
= 
− 
B 1 1
0.75 0.5
[ ] [ ] 0.25 0.5
0.25 0.5
T
f
− −
− − 
 = = = − −
 − 
DCPTDF S X A B 
3.1.2 Sensitivity factors ACPTDF 
The final results of bus voltages are 1 2 31,05; 1,05 0,0771; 1,07 0,0399U U U= = − = − 
Jacobian matrix: 
2 2 2 2
2 3 2 3
3 3 3 3
2 3 2 3
2 2 2 2
2 3 2 3
3 3 3 3
2 3 2 3
10.99 5.13 40.49 20.03
3.52 6
.
.11 20.71
42.24 21.43 5 7 4.79
21.75 32.46 3.35 6.64
P P P P
U U
P P P P
U U
Q Q Q Q
U U
Q Q Q Q
U U
 
 
 
 
    

−
    
    
    = =
     − −
    − − 
   
−

   
−
−
J
31.36
The sensitivity matrix of line power flow with respect to state variables can be expressed as 
follows: 
TNU Journal of Science and Technology 226(06): 82 - 89 
 87 Email: jst@tnu.edu.vn 
12 12 12 12
2 3 2 3
13 13 13 13
2 3 2 3
23 23 23 23
2 3 2 3
21.465 0 3.711 0
10.886 0 1.611
21.429 21. 0
0
429 3.2 4 4.790
P P P P
U U
P P P P
U U
P P P P
U U
 
 
 
    
    
     = =     
−
− −
−    
    
−
D 
The sensitivity matrix of factors ACPTDF (only consider active power): 
1
0.708 0.477
0.26 0.504
0.253 0.495
. −
 = =
− −
− −
− 
ACPTDF D J 
The differences between the sensitivity factors DCPTDF and ACPTDF are determined 
according to (17) and are shown in Table 3. 
( )
DCPTDF ACPTDF
% .100
ACPTDF
D
−
= (17) 
Table 3. Difference (in percentage) between DCPTDF and ACPTDF for 3-bus system 
ACPTDF DCPTDF ( )%D 
-0.708 -0.477 -0.75 -0.5 -2.583 -1.628 
-0.26 -0.504 -0.25 -0.5 -4.438 -1.588 
0.253 -0.495 0.25 -0.5 -1.419 1.471 
The obtained results from a three-bus system above show that the sensitivity factors PTDF 
with the DC model are very close to that of the AC model (the difference is less than 5%). On 
the other hand, in terms of computational performance, computing ACPTDF is more complex 
when compared to DCPTDF. 
3.2. Wood & Wollenberg six-bus system 
This section analyzes the results obtained with a Wood & Wollenberg six-bus system [13]. 
The first bus is the swing bus. 
The obtained results for linear factors DCPTDF and ACPTDF are illustrated in Table 4 and 
Table 5, respectively. In these tables, the numbers such as (1) in the first row and (1-2) in the first 
column represent the bus and transmission line, respectively. 
Figure 2. The change of line power flow (in MW) for bilateral transaction 
TNU Journal of Science and Technology 226(06): 82 - 89 
 88 Email: jst@tnu.edu.vn 
Table 4. Linear factors DCPTDF 
 2 3 4 5 6 
1-2 -0.4706 -0.4026 -0.3149 -0.3217 -0.4064 
1-4 -0.3149 -0.2949 -0.5044 -0.2711 -0.296 
1-5 -0.2145 -0.3026 -0.1807 -0.4072 -0.2976 
2-3 0.0544 -0.3416 0 -0.1057 -0.1907 
2-4 0.3115 0.2154 -0.379 0.1013 0.2208 
2-5 0.0993 -0.0342 0.0292 -0.1927 -0.0266 
2-6 0.0642 -0.2422 0 -0.1246 -0.41 
3-5 0.0622 0.289 0 -0.1207 0.1526 
3-6 0 0.3695 0 0 -0.3433 
4-5 0 -0.0795 0.1166 -0.1698 -0.0752 
5-6 -0.0565 -0.1273 0 0.1096 -0.2467 
Table 5. Linear factors ACPTDF 
 2 3 4 5 6 
1-2 -0.4457 -0.3887 -0.3054 -0.3198 -0.4003 
1-4 -0.3235 -0.3048 -0.5202 -0.286 -0.3129 
1-5 -0.2214 -0.3056 -0.1873 -0.4174 -0.308 
2-3 0.0618 -0.3674 0.02 -0.1185 -0.2144 
2-4 0.3151 0.2359 -0.367 0.1235 0.243 
2-5 0.1044 0 0.0351 -0.1899 0 
2-6 0.0643 -0.2326 0.0207 -0.1241 -0.402 
3-5 0.0654 0.2616 0.0213 -0.1239 0.1468 
3-6 0 0.363 0 0 -0.3541 
4-5 0 -0.0676 0.1108 -0.1597 -0.0686 
5-6 -0.0594 -0.1304 -0.0198 0.1092 -0.244 
A bilateral contract (1, 3, 4, 30 MW) is implemented between the power plant at node 3 
(source node) and the load at node 4 (sink node) with a transacted power of 30 MW. The purpose 
of implementing this scenario is to calculate and compare the power flow change (values and 
directions) using three approaches, including DCPTDF, ACPTDF, and Repeated Power Flow 
(RPF). The results of power flow change in each line are illustrated in Figure 2. The negative 
values indicate that the actual power flow is in the reserve direction. 
As shown in Figure 2, this bilateral transaction can significantly impact the power flow 
change of lines 2-3, 2-4 and 3-6, while the change of power flow in lines 2-5 and 1-2 is 
considerably low. Furthermore, the obtained outcomes from the ACPTDF-based method are 
closer to the RPF method than that of the DCPTDF-based technique. 
4. Conclusion 
This paper studies both methodologies, namely DCPTDF and ACPTDF, to calculate 
transmission usage of bilateral contracts. The results show that the solution obtained with the 
ACPTDF is more accurate than that of DCPTDF and is very close to the solution obtained with 
the repeated power flow method. These findings provide valuable information for Independent 
System Operators (ISO) and Market Operators (MO) to allocate transmission usage service 
equitably, which plays a vital role in operating electricity markets effectively. 
TNU Journal of Science and Technology 226(06): 82 - 89 
 89 Email: jst@tnu.edu.vn 
REFERENCES 
[1] M. Liu and G. Gross, “Role of distribution factors in congestion revenue rights applications,” IEEE 
Trans. Power Syst., vol. 19, no. 2, pp. 802-810, 2004, doi: 10.1109/TPWRS.2004.826708. 
[2] F. For, “Topological generation and load distribution factors for supplement charge allocation in 
transmission open access,” IEEE Trans. Power Syst., vol. 12, no. 3, pp. 1185-1193, 1997. 
[3] D. K. Ron and A. G. Strbac, “Contributions of individual generators to loads and flows,” IEEE Trans. 
Power Syst., vol. 12, no. 1, pp. 52–60, 1997, doi: 10.1109/59.574923. 
[4] F. F. Wu, Yixin Ni, and Ping Wei, “Power transfer allocation for open access using graph theory-
fundamentals and applications in systems without loop flow,” IEEE Trans. Power Syst., vol. 15, no. 3, 
pp. 923–929, 2000, doi: 10.1109/59.871714. 
[5] A. Kumar and S. C. Srivastava, “AC power transfer distribution factors for allocating power 
transactions in a deregulated market,” IEEE Power Eng. Rev., vol. 22, no. 7, pp. 42-43, 2002, doi: 
10.1109/MPER.2002.1016847. 
[6] C. Barbulescu, S. Kilyeni, G. Vuc, B. Lustrea, R. E. Precup, and S. Preitl, “Software tool for power 
transfer distribution factors (ptdf) computing within the power systems,” Ieee Eurocon 2009, Eurocon 
2009, vol. 5, pp. 517-524, 2009, doi: 10.1109/EURCON.2009.5167681. 
[7] C. Wang, V. Vittal, V. S. Kolluri, and S. Mandal, “PTDF-based automatic restoration path selection,” 
IEEE Trans. Power Syst., vol. 25, no. 3, pp. 1686-1695, 2010, doi: 10.1109/TPWRS.2009.2037820. 
[8] H. Ronellenfitsch, M. Timme, and D. Witthaut, “A Dual Method for Computing Power Transfer 
Distribution Factors,” IEEE Trans. Power Syst., vol. 32, no. 2, pp. 1007-1015, 2017, doi: 
10.1109/TPWRS.2016.2589464. 
[9] M. A. Bhaskar and A. A. Jimoh, “Available transfer capability calculation using PTDF and 
implementation of optimal power flow in power markets,” 2016 IEEE Int. Conf. Renew. Energy Res. 
Appl., vol. 5, pp. 14-17, 2016. 
[10] R. Singh, A. K. Singh, and T. Tyagi, “Locational marginal pricing calculation using PTDF through 
load flow and conventional GSF: A comparative study,” 2018 IEEMA Eng. Infin. Conf. eTechNxT, 
2018, pp. 1-6, doi: 10.1109/ETECHNXT.2018.8385366. 
[11] A. B. Ogundare and I. A. Adejumobi, “Transmission Expansion Planning Using Power Transfer 
Distribution Factor Index,” IEEE PES/IAS PowerAfrica Conf. Power Econ. Energy Innov. Africa, 
PowerAfrica, 2019, pp. 6–11, doi: 10.1109/PowerAfrica.2019.8928898. 
[12] K. Zheng, Q. Chen, Y. Wang, C. Kang, and L. Xie, “Unsupervised Congestion Status Identification 
Using LMP Data,” IEEE Trans. Smart Grid, vol. 12, no. 1, pp. 726-736, 2021, doi: 
10.1109/TSG.2020.3011266. 
[13] G. B. S. Allen, J. Wood, and B. F. Wollenberg, Power Generation, Operation and Control. Wiley, 
2014.