Vibration under variable magnitude moving distributed masses of non-uniform bernoulli–euler beam resting on pasternak elastic foundation

 Vietnam Journal of Mechanics, VAST, Vol.41, No. 1 (2019), pp. 63 – 78
 DOI: https://doi.org/10.15625/0866-7136/12781
 VIBRATION UNDER VARIABLE MAGNITUDE
 MOVING DISTRIBUTED MASSES OF NON-UNIFORM
 BERNOULLI–EULER BEAM RESTING ON PASTERNAK
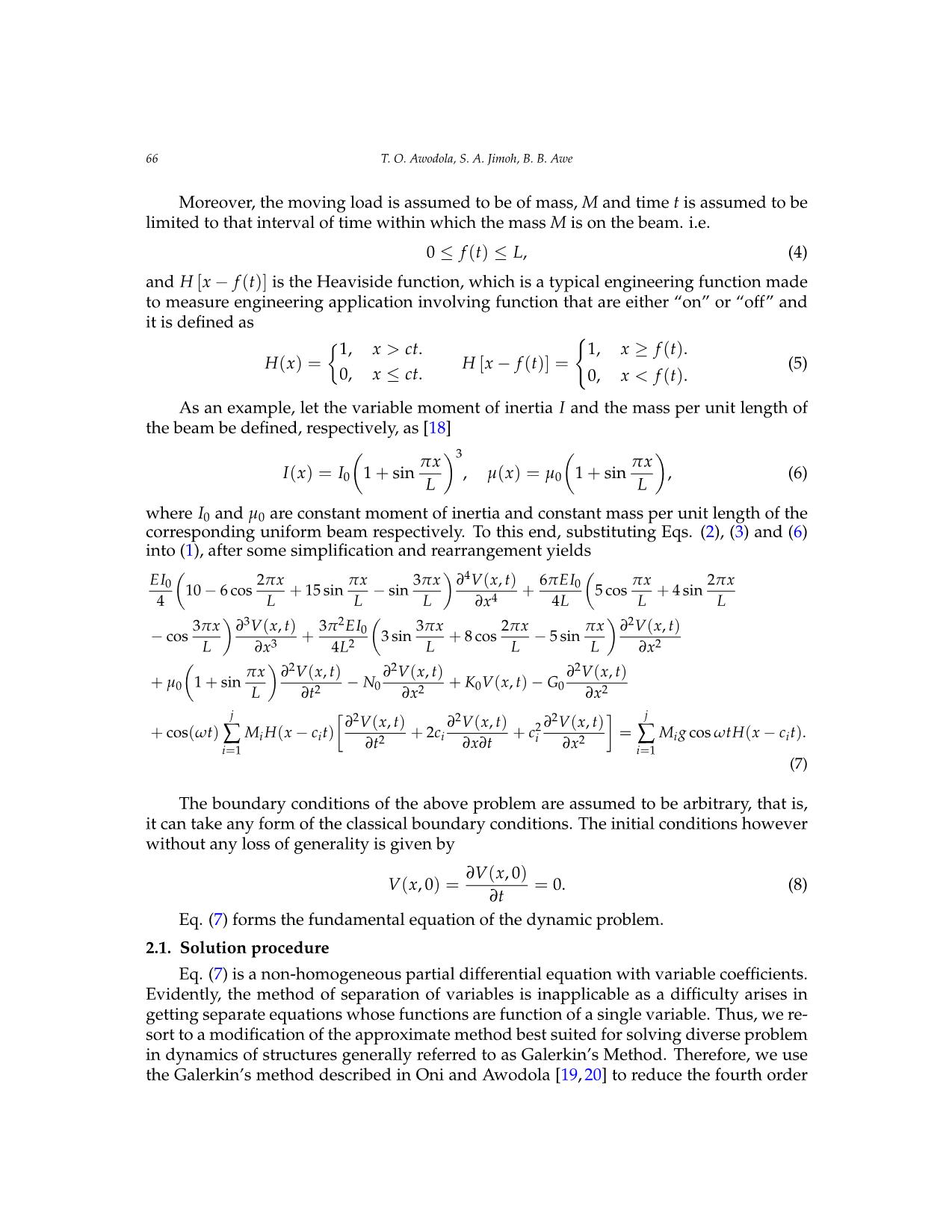
 ELASTIC FOUNDATION
 T. O. Awodola∗, S. A. Jimoh, B. B. Awe
 Federal University of Technology, Akure, Nigeria
 ∗E-mail: toawodola@futa.edu.ng
 Received: 15 July 2018 / Published online: 29 October 2018
 Abstract. The dynamic response to variable magnitude moving distributed masses of
 simply supported non-uniform Bernoulli–Euler beam resting on Pasternak elastic foun-
 dation is investigated in this paper. The problem is governed by fourth order partial dif-
 ferential equation with variable and singular coefficients. The main objective of this work
 is to obtain closed form solution to this class of dynamical problem. In order to obtain
 the solution, a technique based on the method of Galerkin with the series representation
 of Heaviside function is first used to reduce the equation to second order ordinary dif-
 ferential equations with variable coefficients. Thereafter the transformed equations are
 simplified using (i) The Laplace transformation technique in conjunction with convolu-
 tion theory to obtain the solution for moving force problem and (ii) finite element analysis
 in conjunction with Newmark method to solve the analytically unsolvable moving mass
 problem because of the harmonic nature of the moving load. The finite element method
 is first used to solve the moving force problem and the solution is compared with the
 analytical solution of the moving force problem in order to validate the accuracy of the
 finite element method in solving the analytically unsolvable moving mass problem. The
 numerical solution using the finite element method is shown to compare favorably with
 the analytical solution of the moving force problem. The displacement response for mov-
 ing distributed force and moving distributed mass models for the dynamical problem are
 calculated for various time t and presented in plotted curves.
 Keywords: moving mass; finite element; Newmark method; Pasternak elastic foundation;
 Galerkin’s method; resonance.
 1. INTRODUCTION
 Force vibration of elastic bodies (stretched string, spring mass system, rods, etc.)
have been extensively studied by several authors [1–11]. The vibrations may be due to
(i) a force (load) which is a function of the space coordinates only or (ii) a force which
 
c 2019 Vietnam Academy of Science and Technology
64 T. O. Awodola, S. A. Jimoh, B. B. Awe
varies in both space and time. Such forces can either be of constant magnitude or vari-
able magnitude. The present work concerns the effects of a force of variable magnitude
moving at constant speed on an elastic body. In particular, the elastic body under consid-
eration is the beam. It should, however, be mentioned from the onset, that such an elastic
body (long and thin or stubby) is normally considered as a one-dimensional body [9–11]
whose physical properties (stiffness, mass, length) are described with reference to a sin-
gle dimension, the position along the elastic axis. Consequently, the partial differential
equation describing the motion of such an elastic body is made up of only two indepen-
dent variables, distance along the axis and time. Limiting the discussions to that of a
moving force on a finite beam, Timoshenko [2], Inglis [12] and Muscolino [13] considered
the problem of transverse oscillations of a beam subjected to a harmonic force moving
with a uniform velocity. They assumed that the beam is simply supported. An analysis
of the effect of such a moving force on the beam is given. Recently, Steele [14] investigated
the effect of this moving force on beams to a unit force moving at a uniform velocity. The
effect of this moving force on beams with and without an elastic foundation are analyzed.
 Some of the previous works involving non-uniform beams include that of Wu [15]
studied the dynamic responses of multi-span non-uniform beams under moving loads
using the transfer matrix method. Dogush [16] also studied dynamic behavior of multi-
span non-uniform beams traversed by a moving load at constant and variable velocities
using both modal analysis and direct methods. Ahmadian et al. [17] investigated the
analysis of a variable cross-section beam subjected to a moving concentrated force and
mass using finite element method. However, the above research works on both uniform
and non-uniform beams are impactful, but moving loads have been idealized as moving
concentrated loads which act at certain points on the structure and along a single line in
space. That is, the moving load is modelled as a lumped load. In practice, it is known
that loads are actually distributed over a small segment or over the entire length of the
structural member as they traverse the structure. Such moving loads are termed unifo ... p is assembling of the element equations. The procedure for assembling
various matrices and vectors for several beam elements which constitute a mesh is well
discussed in [23, 24]. Hence the governing assembled equation of motion describing the
dynamic behavior of the moving load problem with Pasternak foundation is
 KV(t) + CV˙ (t) + MV¨ (t) = F , (46)
 Vibration under variable magnitude moving distributed masses of non-uniform Bernoulli–Euler beam resting on. . . 73
where K, M, C and F are the assembled (global or overall) stiffness, mass, cen-
tripetal and load vector.
 In order to obtain a complete and unique solution (46), the prescribed boundary con-
ditions must be imposed on both the deflection/slopes and the shear force/bending mo-
ments, respectively. Finally, for a harmonic free vibration system without the centripetal
matrix, (46) reduces to
   2  
 K − ωi M V(t) = 0, (47)
where ω2 denotes the natural frequency and V(t) is the corresponding mode shape of
 2
the system. Various methods can be used to solve for the eigenvalue ωi and the cor-
responding V(t) . The dynamic response of the non-uniform beam under a partially
distributed moving load are obtained by solving the equation of motion (46) directly by
Newmark method.
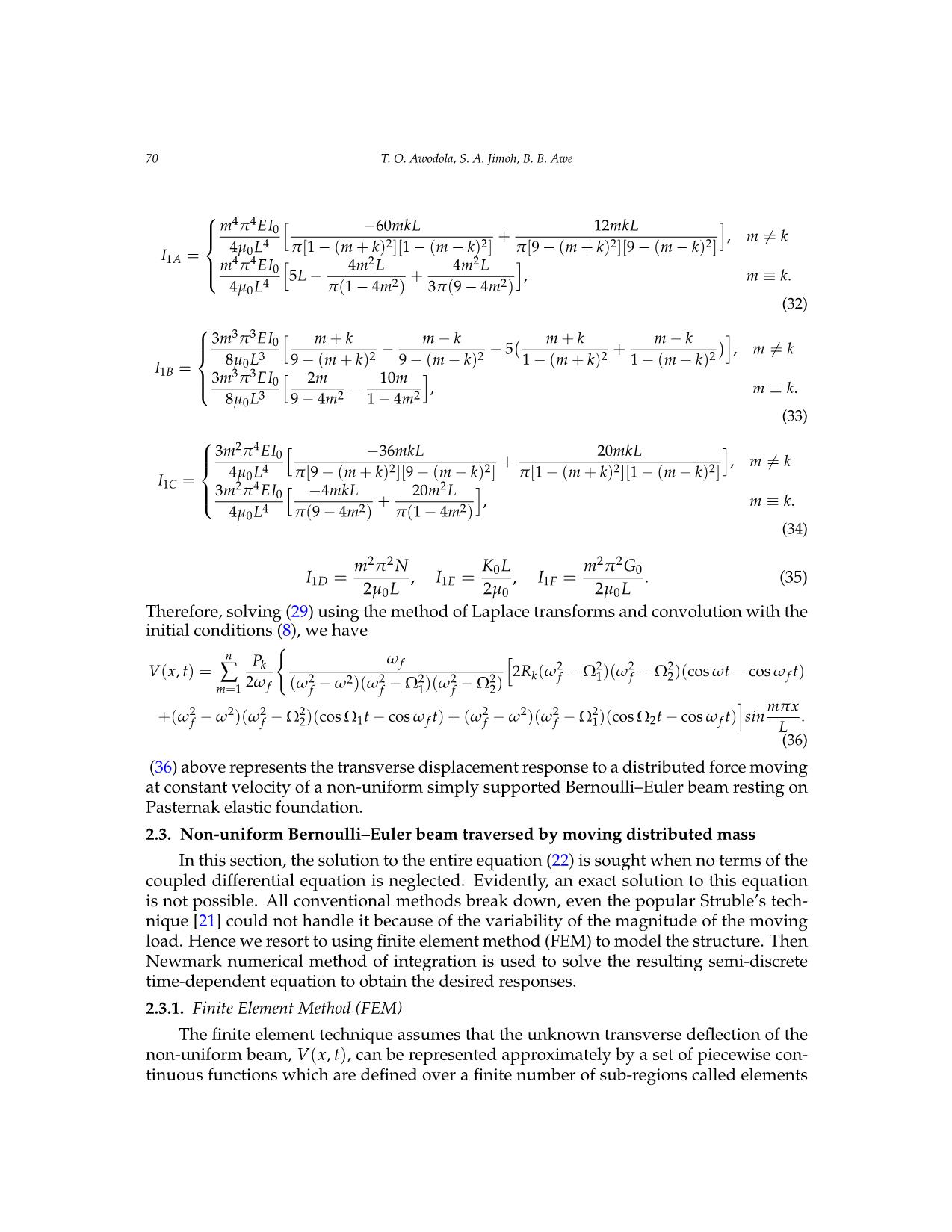
2.3.2. Newmark beta method algorithm
 (a) Initial Computation
 (1) Form stiffness [K], mass [M], and damping [C] matrices
 (2) Initialize {V0}, {V˙0} and {V¨0}
 (3) Select time step ∆t, parameters α and β, and calculate integration con-
 stants.
 β ≥ 0.5; α ≥ 0.25(0.5 + β)2
 1 α 1 1 ∆t α
 a = ; a = ; a = ; a = − 1; a =  − 2
;
 0 β(∆t)2 1 β∆t 2 β∆t 3 2β 5 2 β
 a6 = ∆t(1 − β); a7 = β∆t
 (4) Form effective stiffness matrix:
 [K¯] = [K] + a0[M] + a1[C]
 (5) Triangularize [K¯]: [K¯] = [L][D][L]T
 (b) For each time step:
 (1) Calculate effective force vector at time t + ∆t
  

 {F¯t+∆t} = {Ft+∆t} + [M] a0{Vt} + a2{V˙t} + a3{V¨t}
  

 + [C] a1{Vt} + a4{V˙t} + a5{V¨t}
 (2) solve displacement at time t + ∆t
 [K¯]{Vt+∆t} = {F¯t+∆t}
 (3) calculate {V˙ } and {V¨ } at time t + ∆t
  

 •{ V¨t} = a0 {Vt+∆t} − {Vt} − a2{V˙t} − a3{V¨t}
  

 •{ V˙t} = a1 {Vt+∆t} − {Vt} − a4{V˙t} − a5{V¨t}
74 T. O. Awodola, S. A. Jimoh, B. B. Awe
 3. COMMENTS ON THE CLOSED FORM SOLUTIONS
 Theoretically speaking, the deflections of the non-uniform Bernoulli–Euler beam
may increase beyond bounds. Practically, this means that the beam is in a state of reso-
nance. The speed of the load which brings about resonance effect in the system is termed
the critical speed. (36) clearly shows that the simply supported non-uniform Bernoulli–
Euler beam resting on a Pasternak foundation and traversed by a moving distributed
force reaches a state of resonance whenever
 ω f = ω or ω f = Ω1 or ω f = Ω2. (48)
Eq. (30) shows that, the dynamic system will attain the state of resonance whenever ve-
locity is
 L L
 c = ω − ω 
 or c = ω − ω
. (49)
 mπ f mπ f
Thus, Eq. (49) is critical speed for the dynamic problem.
 4. ANALYSIS OF RESULT AND DISCUSSION
 In order to illustrate the analysis presented in this work, non-uniform beam of length
L = 5 m is considered. The load velocity c = 50 ms−1, Young modulus E = 2.10924 ∗ 109
Nm−2, moment of inertia I = 0.00287698 m4, π = 22/7, mass per unit length of the
beam µ = 2758.291 Kgm−1 and ratio of the mass of the load to the mass of the beam
is 0.25. The transverse deflection of the beam is calculated and plotted against time for
various values of axial force N, foundation stiffness K and shear modulus G. Values of N
are varied between 4E+03 and 4E+09 while the values of K varies from 4E+03 to 4E+09.
The values of G are varied from 4E+03 to 4E+09 N/m3. The results are as shown on the
various graphs in Figs2–7.
Fig. 2. Transverse displacement of the non- Fig. 3. Transverse displacement of the non-
uniform simply supported Bernoulli–Euler uniform simply supported Bernoulli–Euler
beam for various values of axial force N and beam for various values of foundation stiffness
fixed values of K(40000) and G(40000) that tra- K and fixed values of G(40000) and N(40000)
 versed by moving distributed force that traversed by moving distributed force
 Vibration under variable magnitude moving distributed masses of non-uniform Bernoulli–Euler beam resting on. . . 75
Fig. 4. Transverse displacement of the non- Fig. 5. Transverse displacement of the non-
uniform simply supported Bernoulli–Euler uniform simply supported Bernoulli–Euler
beam for various values of shear modus G and beam for various values of axial force N and
fixed values of N(40000) and K(40000) that tra- fixed values of K(4000) and G(4000) that tra-
 versed by moving distributed force versed by moving distributed mass
Fig. 6. Transverse displacement of the non- Fig. 7. Transverse displacement of the non-
uniform simply supported Bernoulli–Euler uniform simply supported Bernoulli–Euler
beam for various values of foundation stiffness beam for various values of shear modus G and
K and fixed values of G(4000) and N(4000) that fixed values of N(4000) and K(4000) that tra-
 traversed by moving distributed mass versed by moving distributed mass
 Figs.2–4 show the transverse displacement responses of a non-uniform simply sup-
ported Bernoulli–Euler beam under distributed moving load travelling at constant ve-
locity under the action of moving distributed force for various values of (i) axial force
N and for fixed values of other parameters; (ii) various values of foundation stiffness K
and for fixed values of other parameters and (iii) various values of shear modulus G and
for fixed values of other parameters. The result shows that as N, K and G increases, the
deflection of the beam decreases. Similar results are obtained when the beam is subjected
to moving mass as shown in Figs.5–7.
76 T. O. Awodola, S. A. Jimoh, B. B. Awe
Fig. 8. Comparison of the transverse displace- Fig. 9. Comparison of the transverse displace-
ment of the moving distributed mass and mov- ment of the analytical and FEM solutions for
ing distributed force for the non-uniform sim- the non-uniform simply supported Bernoulli–
 ply supported Bernoulli–Euler beam Euler beam
Fig. 10. Comparison of the transverse displacement of the moving distributed mass and moving
 distributed with SEM force for the non-uniform simply supported Bernoulli–Euler beam
 Different comparisons of the transverse displacements are shown in Figs.8–10. In
order to verify the accuracy of the present method, the vibration under variable mag-
nitude moving distributed masses of a simply supported non-uniform Bernoulli–Euler
beam resting on Pasternak elastic foundation obtained by the present method and the
frequency-domain spectral element method (SEM) are compared at two different veloc-
ities in Fig. 10. The results show that the dynamic responses obtained by the present
method are almost identical to those obtained by using the SEM.
 5. CONCLUSION
 The vibration under variable magnitude moving distributed masses of simply sup-
ported non-uniform Bernoulli–Euler beam resting on Pasternak elastic foundation is in-
vestigated. The problem is governed by fourth-order partial differential equations with
variable and singular coefficients. The main objective of this work is to obtain closed-
form solution to this class of dynamical problem. In particular, the simply supported
non-uniform Bernoulli–Euler beam is considered. In this dynamical problem, the beam
is not uniform but varied along the span of the beam and as such, the solution to the
governing equation are generally not obtainable by finite integral transform. Conse-
quently, an approach generally used in solving dynamical problem called Galerkin’s
 Vibration under variable magnitude moving distributed masses of non-uniform Bernoulli–Euler beam resting on. . . 77
method is used to transform the governing equation with singular and variable coeffi-
cients. The resulting Galerkin’s equations are thereafter solved using (i) The method of
Laplace transformation and convolution theory to obtain the analytical solutions of the
one-dimensional dynamical problem for moving force problem and (ii) finite element
analysis in conjunction with Newmark method for the case of the moving mass problem
which is analytically unsolvable because of the harmonic nature of the moving load. To
verify the accuracy of the present method used in (i), the dynamic responses of a sim-
ply supported non-uniform Bernoulli–Euler beam obtained by the finite element method
(FEM) are compared in Fig.9 and by frequency-domain spectral element method (SEM)
in Fig. 10. The analytical solutions obtained are analyzed and resonance conditions for
the problems started. Numerical analysis are carried out and the study exhibits the fol-
lowing interesting features:
 1. As the values of axial force increases, the displacement amplitude of the simply
supported non-uniform Bernoulli–Euler beam under the action of moving uniformly dis-
tributed force decreases for fixed shear modulus G and foundation stiffness K. The same
results and analyses obtained for moving mass case.
 2. When the axial force N and shear modulus G are fixed, the displacement of a
simply supported non-uniform Bernoulli–Euler beam resting on Pasternak foundation
and traversed by moving distributed force decreases as the foundation stiffness increase
in the dynamical problem. Similar results and analyses are obtained for the moving mass
case.
 3. For fixed axial force N, foundation stiffness K, the response amplitude of the
simply supported non-uniform Bernoulli–Euler beam under the action of moving force
decreases as the shear modulus G is increased. Similar results and analyses are obtained
for moving mass case. Finally, this research work has suggested valuable methods of
solutions to this class of dynamical problem involving simply supported non-uniform
Bernoulli–Euler beam under variable magnitude moving distributed masses.
 REFERENCES
 [1] A. N. Krylov. Mathematical collection of the papers of the Academy of Sciences, Vol. 61. Piterburg,
 (1905).
 [2] S. P. Timoshenko. On the correction for shear of the differential equation for transverse vibra-
 tions of prismatic bars. The London, Edinburgh, and Dublin Philosophical Magazine and Journal
 of Science, 41, (245), (1921), pp. 744–746. https://doi.org/10.1080/14786442108636264.
 [3] J. T. Kenney. Steady-state vibrations of beam on elastic foundation for moving load. Journal
 of Applied Mechanics, 21, (1954), pp. 359–364.
 [4] M. M. Stanisic, J. C. Hardin, and Y. C. Lou. On the response of the plate to a multi-masses
 moving system. Acta Mechanica, 5, (1), (1968), pp. 37–53. https://doi.org/10.1007/bf01624442.
 [5] S. Sadiku and H. H. E. Leipholz. On the dynamics of elastic systems with
 moving concentrated masses. Ingenieur-Archiv, 57, (3), (1987), pp. 223–242.
 https://doi.org/10.1007/bf02570609.
 [6] S. T. Oni and S. N. Ogunyebi. Dynamical analysis of a prestressed elastic beam with
 general boundary conditions under the action of uniform distributed masses. Jour-
 nal of the Nigerian Association of Mathematical Physics, 12, (1), (2008), pp. 103–120.
 https://doi.org/10.4314/jonamp.v12i1.45492.
78 T. O. Awodola, S. A. Jimoh, B. B. Awe
 [7] S. T. Oni and B. Omolofe. Flexural motions under accelerating loads of structurally pre-
 stressed beams with general boundary conditions. Latin American Journal of Solids and Struc-
 tures, 7, (3), (2010), pp. 285–306. https://doi.org/10.1590/s1679-78252010000300004.
 [8] E. Esmailzadeh and M. Ghorashi. Vibration analysis of beams traversed by uniform par-
 tially distributed moving masses. Journal of Sound and Vibration, 184, (1), (1995), pp. 9–17.
 https://doi.org/10.1006/jsvi.1995.0301.
 [9] S. T. Oni. On thick beams under the action of a variable traveling transverse load, Abacus.
 Journal of Mathematical Association of Nigeria, 2, (1997), pp. 531–546.
[10] M. M. Stanisic and J. C. Hardin. On the response of beams to an arbitrary number of
 concentrated moving masses. Journal of the Franklin Institute, 287, (2), (1969), pp. 115–123.
 https://doi.org/10.1016/0016-0032(69)90120-3.
[11] M. Stanisic, J. A. Euler, and S. T. Montgomery. On a theory concerning the dynamical be-
 havior of structures carrying moving masses. Ingenieur-Archiv, 43, (5), (1974), pp. 295–305.
 https://doi.org/10.1007/bf00537218.
[12] C. E. Sir Inglis. A mathematical treatise on vibrations in railway bridges. Cambridge University
 Press, (1934).
[13] G. Muscolino and A. Palmeri. Response of beams resting on viscoelastically damped foun-
 dation to moving oscillators. International Journal of Solids and Structures, 44, (5), (2007),
 pp. 1317–1336. https://doi.org/10.1016/j.ijsolstr.2006.06.013.
[14] C. R. Steele. Beams and shells with moving loads. International Journal of Solids and Structures,
 7, (9), (1971), pp. 1171–1198. https://doi.org/10.1016/0020-7683(71)90060-6.
[15] J. S. Wu and C. W. Dai. Dynamic responses of multispan nonuniform beam due
 to moving loads. Journal of Structural Engineering, 113, (3), (1987), pp. 458–474.
 https://doi.org/10.1061/(asce)0733-9445(1987)113:3(458).
[16] Y. Dugush and M. Eisenberger. Vibrations of non-uniform continuous beams un-
 der moving loads. Journal of Sound and Vibration, 254, (5), (2002), pp. 911–926.
 https://doi.org/10.1006/jsvi.2001.4135.
[17] M. Ahmadian, E. Esmailzadeh, and M. Asgari. Dynamic analysis of non-uniform cross sec-
 tions beam under moving mass using finite element method. In Proceedings of the 4th CSME
 Forum, Calgari, Alberta, Canada, May 21st-23rd, (2006), pp. 384–395.
[18] S. T. Oni. On the dynamic response of elastic structure to moving multi-mass system. PhD thesis,
 University of Ilorin, Nigeria, (1991).
[19] S. T. Oni and T. O. Awodola. Vibrations under a moving load of a non-uniform Rayleigh
 beam on variable elastic foundation. Journal of Nigerian Association of Mathematical Physics, 7,
 (2003), pp. 191–206.
[20] S. T. Oni and T. O. Awodola. Dynamics behavior under moving concentrated masses of elas-
 tically supported finite Bernoulli–Euler beam on Winkler foundation. Latin American Journal
 of Solid and Structures, 7, (1), (2010), pp. 2–20.
[21] R. Struble. Nonlinear differential equation. New York, San-Francisco, Toronto, London, Sydney,
 (1962).
[22] J. L. Junkins and Y. Kim. Introduction to dynamics and control of Flexible structure. American
 Institute of Aeronautic and Astronautic, Inc. Washington, DC, (1993).
[23] J. J. Wu. Vibration analyses of a portal frame under the action of a moving distributed mass
 using moving mass element. International Journal for Numerical Methods in Engineering, 62,
 (14), (2005), pp. 2028–2052. https://doi.org/10.1002/nme.1268.
[24] T. Irvine. Transverse vibration of a beam via the finite element method. Revision E, Vibration data,
 Citeseer, (2010).