Optimal parameters of linear dynamic vibration absorber for reduction of torsional vibration

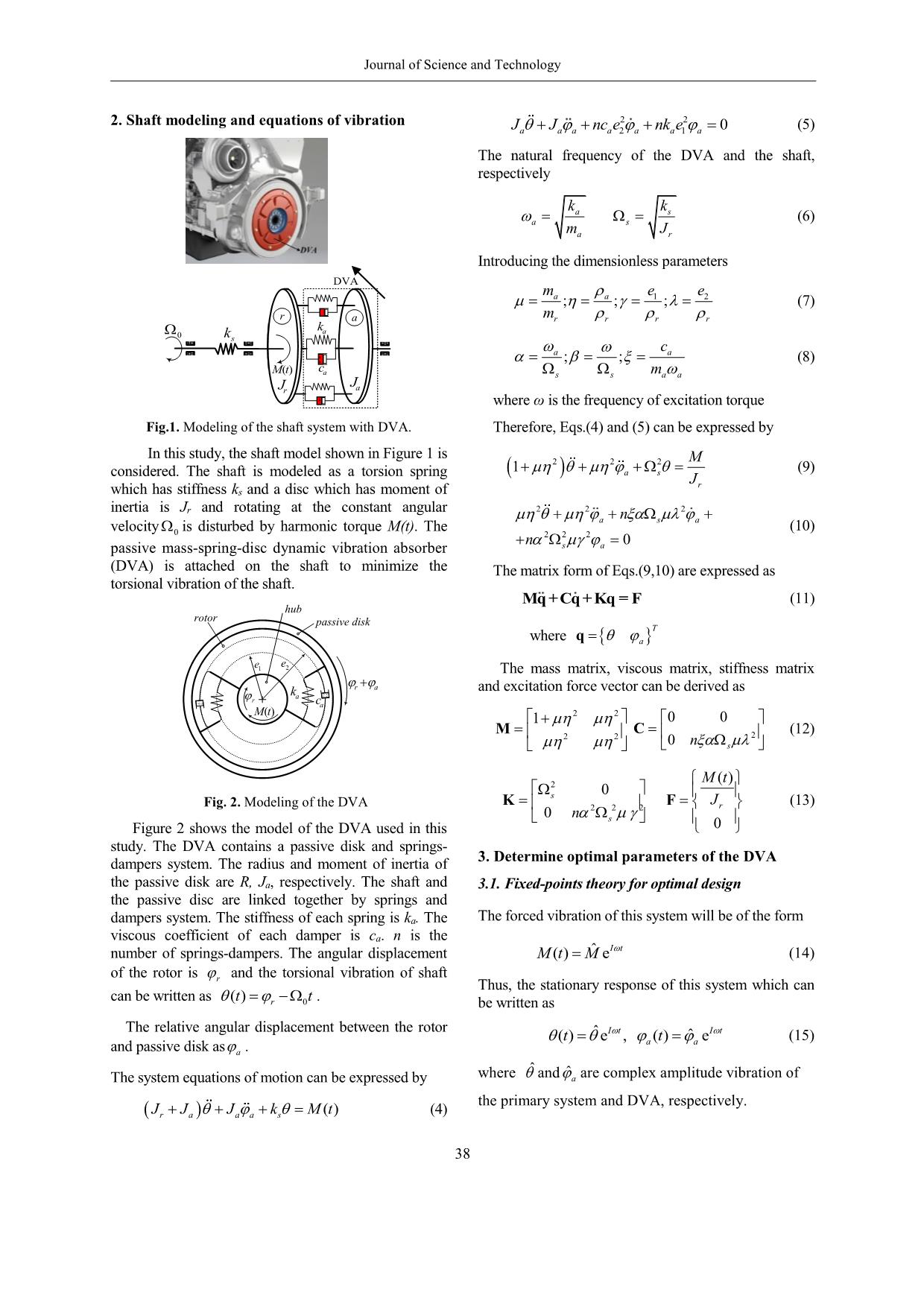
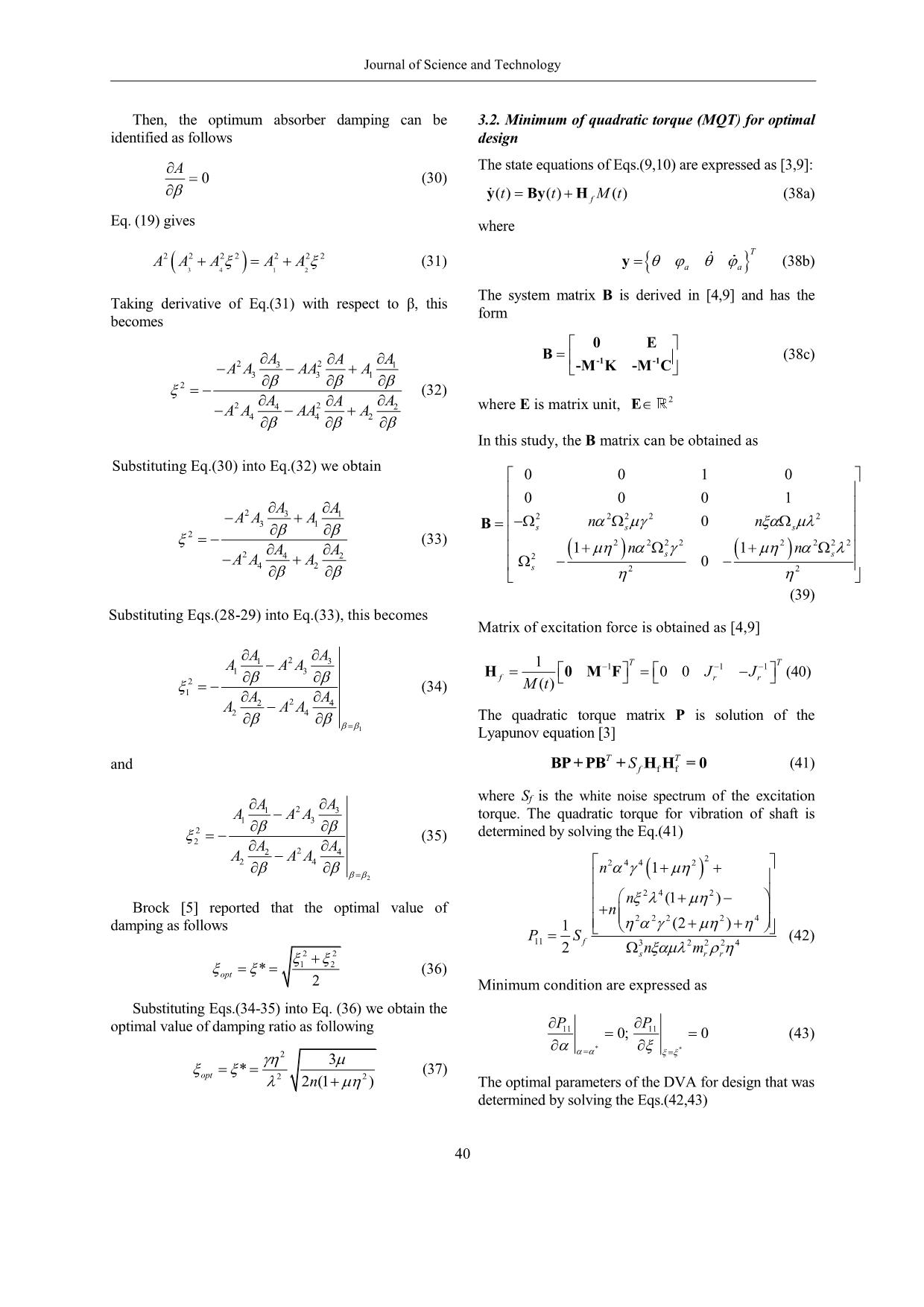
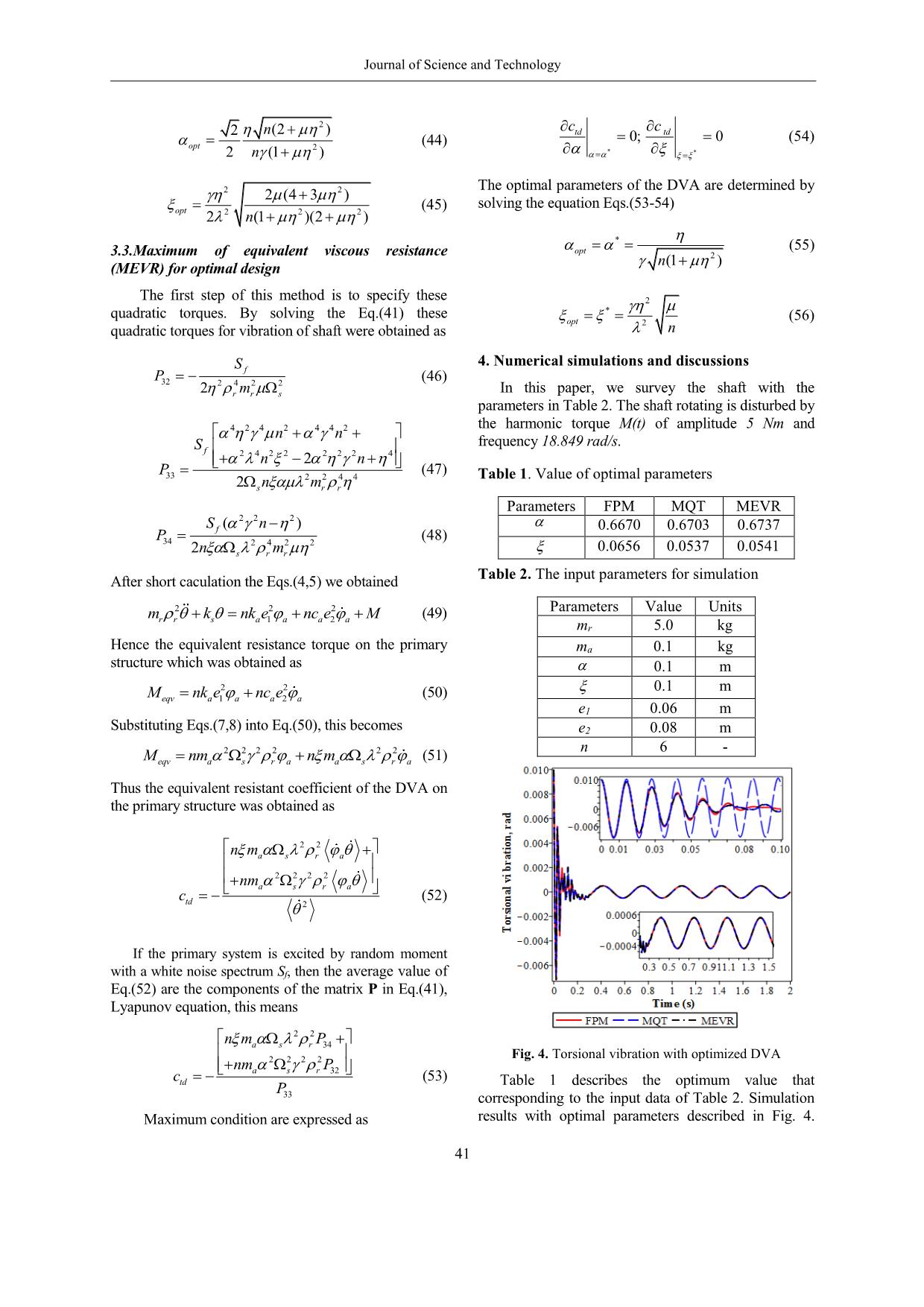
 Journal of Science & Technology 119 (2017) 037-042 
 Optimal Parameters of Linear Dynamic Vibration Absorber for Reduction 
 of Torsional Vibration 
 Vu Xuan Truong1, 2, Khong Doan Dien2, Nguyen Duy Chinh2, Nguyen Duc Toan 3,* 
 1 Graduate University of Science and Technology, Vietnam Academy of Science and Technology, Hanoi, Vietnam 
 2 Faculty of Mechanical Engineering, Hungyen University of Technology and Education, Hungyen, Vietnam 
 3 School of Mechanical Engineering, Hanoi University of Science and Technology, Hanoi, Vietnam 
 Received: October 24, 2016; accepted: June 9, 2017 
 Abstract 
 This paper presents three analytical methods to determine optimal parameters of the passive mass-spring-
 disc dynamic vibration absorber (DVA), such as the ratio between natural frequency of DVA and shaft, damping 
 ratio of DVA. The original model presented by Den Hartog, Luft and Warburton are solved and has shown in 
 good agreement. Three analytical methods is then adopted for torsional shaft model. The simulation results 
 indicate that the effectiveness in torsional vibration could be reduced. Finally, the optimal parameters of DVA 
 were applied to decrease the shaft torsional vibration considering the vibration duration and stability criterion. 
 Keywords: Dynamic vibration absorber, Torsional vibration, Fixed-points theory. 
1. Introduction where  is the ratio of the absorber’s mass to the 
 The*dynamic vibration absorber (DVA) or tuned- primary structure’s mass. 
mass damper (TMD) is a widely used passive vibration Since then, the fixed-points theory and DVA 
control device. When a mass-spring system, referred to structures have become one of the design laws used in 
as primary system, is subjected to a harmonic excitation optimizing design of the damped and undamped 
at a constant frequency, its steady-state response can be primary system [6-8]. 
suppressed by attaching a secondary mass-spring Luft proposed methodology MEVR (maximum of 
system or DVA. This idea was pioneered by Watts in equivalent viscosity resistance) for the original model 
1883 and Frahm in 1909. However, a DVA consisting [2]. Later, Warburton used minimum of quadratic 
of only a mass and spring has a narrow operation region torque method (MQT) and found that the damping in 
 the neutralizer can also be optimized [3]. The results 
and its performance deteriorates significantly when the 
 was given by 
exciting frequency varies. The performance robustness 
can be improved by using a damped DVA that consists 1/ 2 (13/ 4)
  ; (3) 
of a mass, spring, and damper. The key design optopt 14(1/  2)(1)
parameters of a damped DVA are its tuning parameter 
and damping ratio. This paper presents three analytical methods such 
 as FPM, MQT and MEVR to determine optimal 
 The optimization technique for original model that parameters of the dynamic vibration absorber (DVA) 
is described in detail by Den Hartog [1]. The optimum for new shaft model such as the ratio between natural 
tuning ratio of the neutralizer was found as a function of frequency of DVA and shaft, the damping ratio of 
the neutralizer’s mass given by absorber. Since then we compare and evaluate optimal 
 effectiveness of those methods. Based on the main idea 
 1
 (1) is to build a program that calculates to prove optimal 
 opt 1  analytic solution of the original model, which applies to 
and the damping ratio of the absorber torsion shaft model. Optimal parameters are presented 
 3 as very neat analysis. The simulation results indicate 
  (2) that the effectiveness in torsional vibration reduction. 
 opt 81  
* Corresponding author: Tel.: (+84) 988.693.047 
Email: toan.nguyenduc@hust.edu.vn 
 37 
 Journal of Science and Technology 
2. Shaft modeling and equations of vibration 22
 JJncenkeaaaaaaa 210 (5) 
 The natural frequency of the DVA and the shaft, 
 respectively 
 ka ks
 a  s (6) 
 ma Jr
 Introducing the dimensionless parameters 
 DVA
 m ee
  aa;;; 12 (7) 
 r a mrrrr 
  ka
 0 ks
   c
  aa;; (8) 
 c
 Mt() a ssaa m 
 J
 Jr a
 where ω is the frequency of excitation torque 
 Fig.1. Modeling of the shaft system with DVA. Therefore, Eqs.(4) and (5) can be expressed by 
 In this study, the shaft model shown in Figure 1 is 
 222 M
considered. The shaft is modeled as a torsion spring 1    as (9) 
 Jr
which has stiffness ks and a disc which has moment of 
inertia is Jr and rotating at the constant angular    222  n
velocity  is disturbed by harmonic torque M(t). The asa (10) 
 0  n  222 0
passive mass-spring-disc dynamic vibration absorber sa
(DVA) is attached on the shaft to minimize the The matrix form of Eqs.(9,10) are expressed as 
torsional vibration of the shaft. 
 Mq +Cq +Kq = F (11) 
 hub
 rotor passive disk
 T
 where q  a  
 e e
 1 2 The mass matrix, viscous matrix, stiffness matrix 
 ra and excitation force vector can be derived as 
 ka
 r
 ca
 Mt() 1 22  00
 C 
 M 22 2 (12) 
   0 n s
 Mt()
 2 0 
 s J
 Fig. 2. Modeling of the DVA K 2 2 2 F r (13) 
 0 n s  
 Figure 2 shows the model of the DVA used in this 0
study. The DVA contains a passive disk and springs-
dampers system. The radius and moment of inertia of 3. Determine optimal parameters of the DVA 
the passive disk are R, Ja, respectively. The shaft and 3.1. Fixed-points theory for optimal design 
the passive disc are linked together by springs and 
dampers system. The stiffness of each spring is ka. The The forced vibration of this system will be of the form 
viscous coefficient of each damper is ca. n is the 
 ˆ It
number of springs-dampers. The angular displacement M( t ) M e (14) 
of the rotor is and the torsional vibration of shaft 
 r Thus, the stationary response of this system which can 
can be written as  ()tt  . 
 r 0 be written as 
 The relative angular displacement between the rotor ˆ I t I t
 (tt )  e , aa ( ) ˆ e (15) 
and passive disk as a . 
 ˆ ˆ
The system equations of motion can be expressed by where  and a are complex amplitude vibration of 
 the primary system and DVA, respectively. 
 Jr J a  J a a k s  M() t (4) 
 38 
 Journal of Science and Technology 
Substituting Eqs.(14-15) into Eq.(11), this becomes some of damping ratio. For c = 0 or c becomes infinite 
 so the amplifier fuction curve becomes infinite. That 
 means some where in between there must be a value of 
 22
 2 1  damping ratio for which the peak becomes a minimum. 
  22
  Two other curves are draw in Fig. 3, for ξ = 0.1 and 0.4. 
 00 ˆ 1 Mˆ The first step of this method is to specify two 
 2i (16) 
 0 n  2 0 fixed points. Suppose that two points (S and T) with 
 s ˆa ks
 horizontal coordinates as a β1, β2. The conditions for A 
 2
 s 0 does not depend on the damping ratio is expressed as 
 222 follows 
 0 n s
 A
 0 (20) 
 Hence the stationary response of the primary 
system is expressed as 
 Substituting Eq.(19) into Eq.(20), this becomes 
 A iA  Mˆ
 ˆ 12 (17) 
 A iA k ()AAAA2222 
 34s 1423 0, (21) 
 222
 2 AA 
 AA222 12
where 43 222
 AA34 
 2 2 2 2 2
 An1    ; An2   ; 2222
 AAAA1423 0 (22) 
 Annn  2222222422222   
 3 From Eq.(22), we have 
 322322
 Annn4    
 AA
 12 (23) 
 After short caculation the Eq.(17) we obtained the  11
 AA34
real amplitude of the vibration response, which can be 
written as 
 AA
 12 (24) 
 AA222  MMˆˆ AA 22
 ˆ()tA 12 (18) 34
 AA222  kk
 34 ss We obtain the value of A at two points (S, T) these 
 where A is called the amplifier function that is are expressed as follows 
defined by 
 A2
 222 A (25) 
 AA  S  1
 12 A4
 A 222 (19) 
 AA34 
 A2
 A T (26) 
 A  2
  4
  1 Den Hartog [1] reported that the graph of amplifier 
 function does not change in between the two peaks (S, 
  0 T) when the vertical coordinates of the S and T must be 
 equal. In this condition, we have 
  0  0.4 AAST (27) 
 The optimal parameter of α and β are specifed by 
 T  0.1
 S solving Eqs.(23-27) which can be written as 
 
 * (28) 
 n  2 1 
 Fig. 3 The graph of amplifier function 
 2 * 2  1
 1,2  1,2  22 (29) 
 Figure 3 shows a plot of the amplifier fuction with  21 
 39 
 Journal of Science and Technology 
 Then, the optimum absorber damping can be 3.2. Minimum of quadratic torque (MQT) for optimal 
identified as follows design 
 A The state equations of Eqs.(9,10) are expressed as [3,9]: 
 0 (30) 
 
 yByH()()()ttMt f (38a) 
Eq. (19) gives where 
 T
 AAAAA2 2 2 2 2 2 2 (31) y   (38b) 
 3 4 1 2 aa
 The system matrix B is derived in [4,9] and has the 
Taking derivative of Eq.(31) with respect to β, this 
 form 
becomes 
 0E
 A A A B -1-1 (38c) 
 A22 A3 AA A 1 -MK-MC
 3 3   1  
  2 (32) 
 AAA 2
 A22 A42 AA A where E is matrix unit, E 
 4 4   2  
 In this study, the B matrix can be obtained as 
Substituting Eq.(30) into Eq.(32) we obtain 
 0010
 0001
 A A 
 AAA2 3 1 22222
 31 B sss nn   0
 2 
  (33) 22222222 
 2 AA 11     nnss 
 AAA 42 2
 42  s 220
  
 (39) 
Substituting Eqs.(28-29) into Eq.(33), this becomes 
 Matrix of excitation force is obtained as [4,9] 
 A1 2 A3
 1 111 TT
 AA13 A 
  H0Mfrr F 00JJ(40) 
  2 (34) Mt () 
 1 AA
 AA A24 2
 24 The quadratic torque matrix P is solution of the 
  1 Lyapunov equation [3] 
 TT
and BP + PB+HS H=f ff 0 (41) 
 where Sf is the white noise spectrum of the excitation 
 A1 2 A3
 AA13 A torque. The quadratic torque for vibration of shaft is 
 2  determined by solving the Eq.(41) 
 2 (35) 
 AA242
 AA A 2
 24 2 4 42
  n  1 
 2 
 n2  42(1) 
 Brock [5] reported that the optimal value of n 
 2 2 224
damping as follows 1  (2) 
 PS11 f 32 2 2 4 (42) 
 22 2 srnm  r 
 12 
 opt * (36) 
 2 Minimum condition are expressed as 
 Substituting Eqs.(34-35) into Eq. (36) we obtain the 
 PP
optimal value of damping ratio as following 1111 0;0 (43) 
   * *
 2 3  
  * (37) 
 opt 222n (1) The optimal parameters of the DVA for design that was 
 determined by solving the Eqs.(42,43) 
 40 
 Journal of Science and Technology 
 2 cc
 2 n(2) td 0; td 0 (54) 
 opt 2 (44) 
 2 n(1)   *  *
 The optimal parameters of the DVA are determined by 
 222(43) 
  (45) solving the equation Eqs.(53 -54 ) 
 opt 2(1)(2)222 n 
 * 
3.3.Maximum of equivalent viscous resistance opt (55) 
 n(1) 2
(MEVR) for optimal design 
 The first step of this method is to specify these 
 2
quadratic torques. By solving the Eq.(41) these *
 opt 2 (56) 
quadratic torques for vibration of shaft were obtained as  n
 4. Numerical simulations and discussions 
 S f
 P32 2 4 2 2 (46) 
 2 rm r  s In this paper, we survey the shaft with the 
 parameters in Table 2. The shaft rotating is disturbed by 
 the harmonic torque M(t) of amplitude 5 Nm and 
 4  2  4 nn 2 4  4 2
 S frequency 18.849 rad/s. 
 f 2 4 2 2 2 2 2 4
 nn  2   
 P33 2 2 4 4 (47) Table 1 . Value of optim al parameters 
 2snm  r r 
 Parameters FPM MQT MEVR 
 222
 Snf ()  0.6670 0.6703 0.6737 
 P34 2422 (48) 
 2nm  srr  0.0656 0.0537 0.0541 
After short caculation the Eqs.(4,5) we obtained Table 2. The input parameters for simulation 
 222 Parameters Value Units 
 mknkrrsaaaa  enc eM 12 (49) 
 mr 5.0 kg 
Hence the equivalent resistance torque on the primary ma 0.1 kg 
structure which was obtained as 0.1 m 
 22  0.1 m 
 Mnkeqvaaaa enc e 12 (50) 
 e1 0.06 m 
Substituting Eqs.(7,8) into Eq.(50), this becomes e2 0.08 m 
 22 2222 n 6 - 
 Mnmneqvasraasra   m    (51) 
Thus the equivalent resistant coefficient of the DVA on 
the primary structure was obtained as 
 nm  22 
 a s r a
 nm 2 2  2 2 
 a s r a
 ctd (52) 
  2
 If the primary system is excited by random moment 
with a white noise spectrum Sf, then the average value of 
Eq.(52) are the components of the matrix P in Eq.(41), 
Lyapunov equation, this means 
 22 
 n ma  s  r P34
 2 2 2 2 Fig. 4. Torsional vibration with optimized DVA 
 nma s  r P32
 ctd (53) Table 1 describes the optimum value that 
 P
 33 corresponding to the input data of Table 2. Simulation 
 Maximum condition are expressed as results with optimal parameters described in Fig. 4. 
 41 
 Journal of Science and Technology 
These results show that torsional vibration of shaft (NAFOSTED) under grant number 107.02-2016.01. 
without DVA has a harmonic form amplitude of about 
 References 
0.02 rad. 
 [1]. Den Hartog J.P., Mechanical Vibrations, 4th Edition, 
 Figure 4 shows that in the first 0.4s, the amplitude McGraw-Hill, NY, (1982). 
of the torsional vibration reduces rapidly. Effectiveness 
of the optimal DVA using FPM is highest in [2]. Luft, R.W., Optimal Tuned Mass Damper for building, 
comparison to the two other methods, however, the J. Struct. Div., ASCE, 105(12) (1979) 2766-2772. 
difference between the viration response curves are [3]. Warburton G.B., Optimum absorber parameters for 
negligible, especially vibration responses of the system various combinations of response and excitation 
with MQT and MEVR are nearly the same. This shows parameters, earthquake Engineering and Structural 
the strength of the fixed-points theory compared to Dynamics, (1982) 381-401. 
other analytical methods. After the above period, the [4]. Khang N.V, Engineering Mechanics, Viet Nam 
torsional vibration of the shaft shifts to the steady state Education Publishing House, (2009). 
with a very small amplitude of about 1.20E-03 rad. At 
 [5]. Brock J.E, A Note on the Damped Vibration Absober, 
this stage, the vibration responses with optimal DVA J. Appl. Mech., 13(4). A-284, 1946 
determined by all methods are almost identical. 
 [6]. Nishihara O, and Asami T, Close-form solutions to the 
5. Conclusions exact optimizations of dynamic vibration absorber 
 In this paper, three analytical methods have been (minimizations of the maximum amplitude 
 manification factors), Journal of Vibration and 
developed and examined for new shaft model. The Acoustics, 124, 576-582, 2002. 
same procedure as in the conventional analytical theory 
has been used to derive the optimum tuning and [7]. Liu K, and Liu J, The damped dynamic vibration 
damping ratios of the device. Research results are absorber: revisited and new result, Journal of Sound 
verified by numerical simulation with high reliability. and Vibration 284, 1181-1189, 2005. 
The optimal parameters were determined in analytical [8]. Anh N.D, and Nguyen N.X, Design of TMD for 
form and furthermore leads to the simple explicit damped linear structures using the dual criterion of 
formulas. The results presented in this paper may offer equivalent linearization method, International Journal 
new ways of using the device over the conventional one. of Mechanical Sciences 77, 164-170, 2013. 
Acknowledgements [9]. Nagashima I., Optimal displacement feedback control 
 law for active tuned mass damper, Earthquake 
 This research is funded by Vietnam National engineering and structural dynamic, 30: 1221-1242, 
Foundation for Science and Technology Development (2001). 
 42